MOOTH
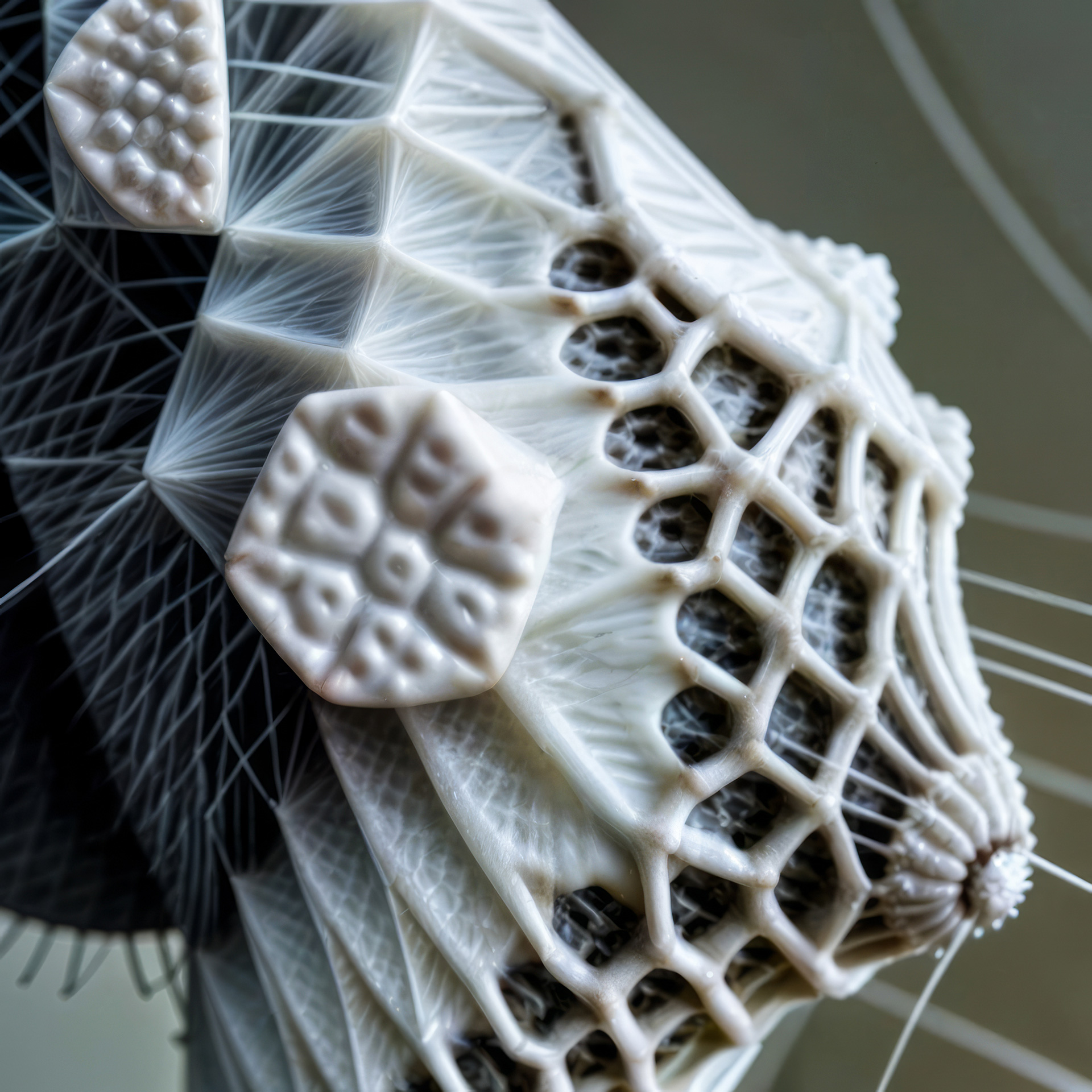
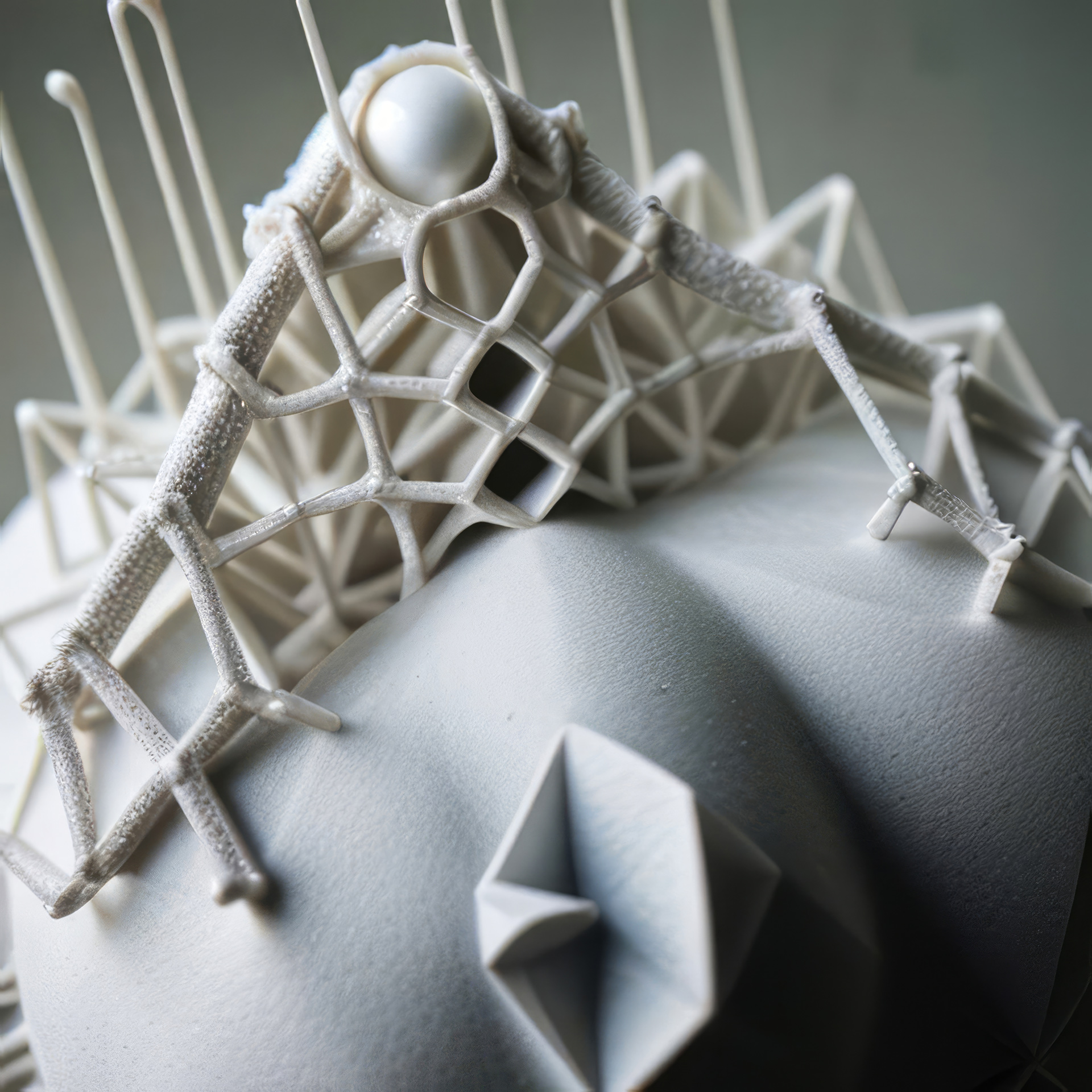
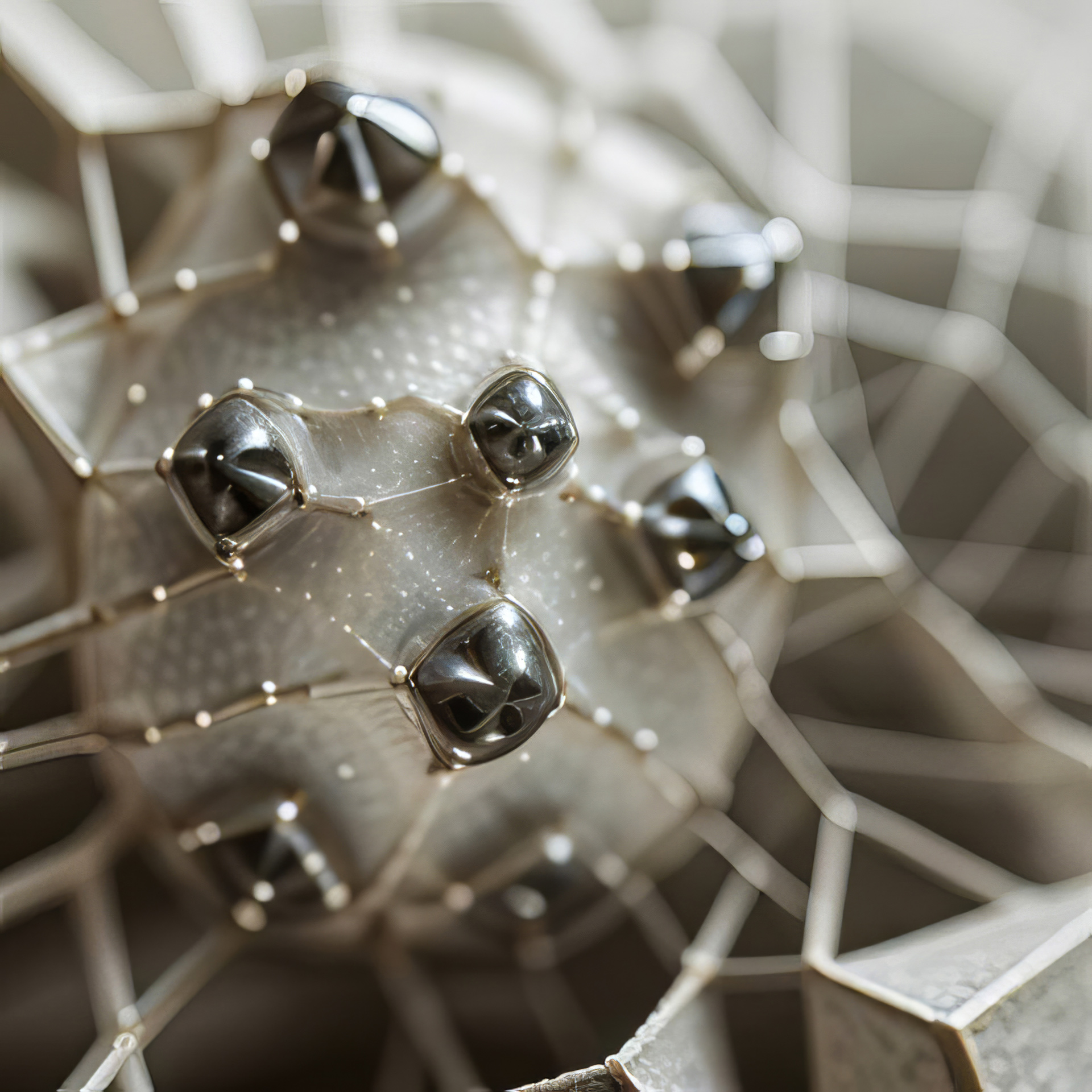
The intersection of fashion, mathematics, and insect structures demonstrates how geometric and biological principles inform textile design. Fractal patterns, observed in dragonfly wings, exemplify complex geometric networks following mathematical sequences such as Fibonacci. These fractal structures, optimized through evolution, translate into textile design to capture the efficiency and intrinsic beauty of natural forms.
The radial symmetry of beetle exoskeletons manifests how mathematics underlie natural shapes. This symmetry, describable through concepts like the golden ratio, applies in fashion design to create structures that are both aesthetically harmonious and functionally robust. Mathematics provides a framework to understand and replicate the interplay between form and function in the natural world.
The hexagonal architecture of bee honeycombs represents optimized spatial efficiency explainable through geometry. Hexagons, maximizing space utilization with minimal material, illustrate a mathematical principle of optimization. In the context of textile design, this hexagonal pattern is employed to blend traditional techniques with modern technologies, creating textiles that reflect the precision and order of natural structures.
| Client: | Personal |
| Date: | 03.07.2024 |
| Share: |